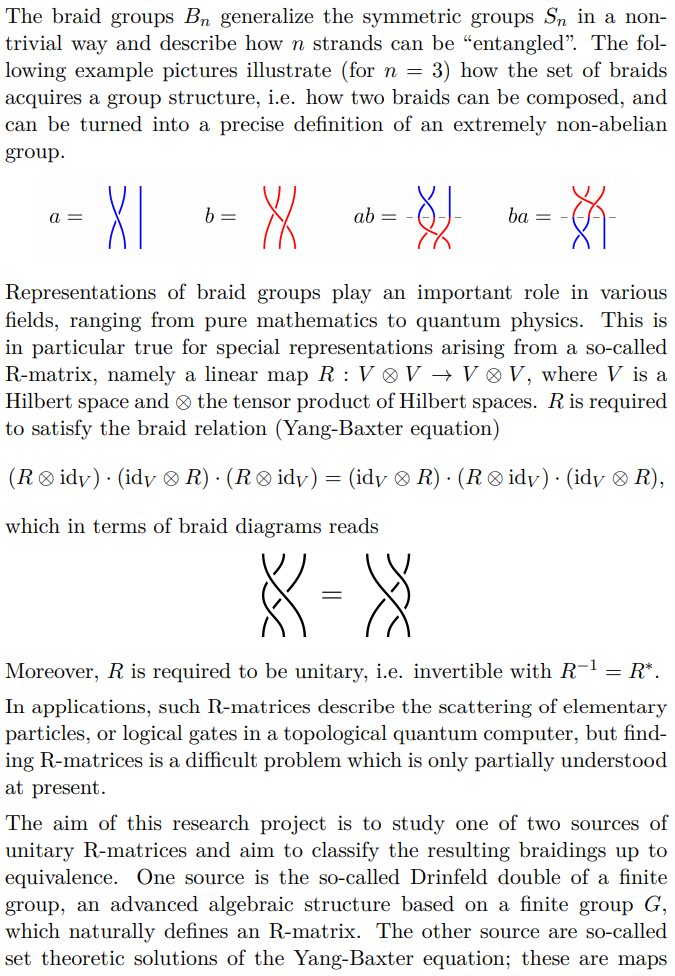
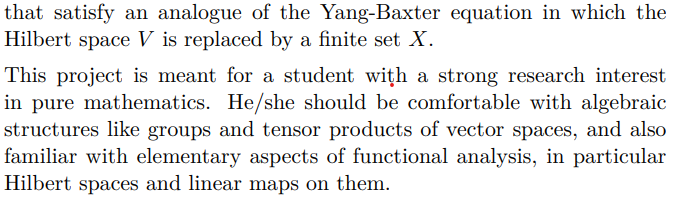
Code: UP2122C

Supervisor: Ulrich Pennig

Project Description:
The fundamental group of a topological space, which we encountered in the algebraic topology course, is defined as the homotopy classes of based loops in the space, where the group multiplication is induced by concatenation. The fundamental group of the torus is generated by two such loops a and b. These commute, i.e. they satisfy ab = ba. Now consider the topological space obtained by gluing together two circles at a chosen base point, which looks like the figure 8. The fundamental group of this space is also generated by two elements, but this time there are no relations among them: The fundamental group is the free group on two generators.

The first goal of this project is to understand the definition of free groups and, more generally, amalgamated products of groups. We will then prove the theorem that subgroups of free groups are again free. This purely algebraic statement has a beautiful proof based on the theory of covering spaces. In the lecture we have already seen how the real line covers the circle in the sense that there is a map to the circle, such that preimages of neighbourhoods look like multiple copies of themselves. During the course of the project we will understand the basics of covering space theory, discuss coverings and fundamental groups of graphs and finally prove the result mentioned above.

Type: Year 4 MMath Project

Prerequisite modules:
– Algebraic Topology (MA3008)
– Groups (MA0213) or Algebra II: Rings (MA3013)

Max. number of students: 1

Title of Project: Dimension Reduction and Classification Algorithms

Code: AA2122A

Supervisor: Dr A. Artemiou

Project description:
Data mining and dimension reduction are two topics which receive great attention in the big data era we are in. There are a number of algorithms which were proposed to discuss data mining algorithms (like Support Vector Machines) and dimension reduction algorithms (like Sufficient Dimension Reduction). Recently, the two areas were combined to create new algorithms for dimension reduction in regression using ideas from the data mining literature. This created a class of new algorithms that perform very well and are able to do linear and nonlinear dimension reduction in a unified framework.
The project objective is to create first new algorithms for data mining by combining existing algorithms. The student will review the given literature and then derive results for new methodology for classification. The performance of the new algorithm will be evaluated using real datasets. Then, the algorithms will be used to perform dimension reduction to regression problems based on existing literature. Good programming skills are essential for this project. (In 2017-2018 a student work on this project and developed a modified version of SVM)

Prerequisite 3rd year module:
MA2500: Foundations of Probability and Statistics;
MA 2501: Programming and Statistics
and MA3505: Multivariate Statistics is helpful but not essential.

Number of students who can be supervised on this project:
1

Title of Project:
Viscosity Solutions and Nonlinear Partial Differential Equations

Code: FD2122B

Supervisor: Dr. F. Dragoni

Project description:
Nonlinear partial differential equations (PDEs) describe many phenomena
in sciences and economics, e.g. the value of an optimal control problem,
minimal surfaces, porous media, conservation laws, etc. Solutions to these
equations are often not sufficiently regular in order to write all the derivatives
appearing in the equation (e.g. they can be just continuous and not
differentiable). So the solutions have to be understood in a suitable generalized
sense. We consider the so-called viscosity solutions which consist
in using sufficiently smooth test functions to approximate from above and
below a unique continuous solution for a very large class of first-order and
second-order PDEs.
A project would consist of an introduction to the general theory of viscosity
solutions and applications to a specific nonlinear PDE (e.g. Hamilton-Jacobi
eq.s, infinite-Laplace eq., p-Laplace eq., etc.). One possibility is to investigate
the relation between viscosity solutions and control theory or games
theory. Another possibility is to focus on degenerate elliptic/parabolic equations
(e.g. the evolution by mean curvature flow) or the relations with convexity.
If required, it will be possible to deal with more geometric equations
(space-dependent) and metric formulas. One possibility is to investigate the
relation between viscosity solutions and control theory or games theory.

Project offered a double module, single module, or both:

Prerequisite 3rdd year modules:
The following classes may be helpful:
MA3303 Theoretical and Computational PDEs

Number of students who can be supervised on this project:
1

Title of project: Unitary Braidings

Code: GL2122A

Supervisor: Dr G. Lechner

Project description:

Project offered as double module, single module, or both: Double

Prerequisite modules: Linear Algebra I+II, Groups, Functional and Fourier Analysis

Number of students who could be supervised for this project: 1

Year: 4

Title of project: Reproducing kernel Hilbert C∗ -modules and applications to machine learning

Code: GL2122B

Supervisors: Dr. B. Gauthier and Dr. G. Lechner

Project Description:

Project offered as double module, single module, or both: Double

Prerequisite modules: Functional and Fourier Analysis

Number of students who could be supervised for this project: 1–2

Year: 4